Question

An air-standard Diesel cycle has a compression ratio of 16 and a cutoff ratio of 2. At the beginning of the compression process, air is at 95 kPa and 278C. Accounting for the variation of specific heats with temperature, determine (a) the temperature after the heat-addition process, (b) the thermal efficiency, and (c) the mean effective pressure

Answer 1

`a.T_3=1723.8kPa\\b.n=0.563\\c.MEP=674.95kPa`

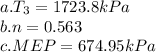
Step-by-step explanation:

a. Internal energy and the relative specific volume at
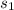`s_1` are determined from A-17:
`u_1=214.07kJ/kg, \ \alpha_r_1=621.2`.

The relative specific volume at
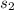`s_2` is calculated from the compression ratio:

`\alpha_r_2=(\alpha_r_1)/(r)\\=(621.2)/(16)\\=38.825`

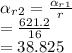
#from this, the temperature and enthalpy at state 2,
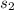`s_2` can be determined using interpolations
`T_2=862K` and
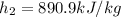`h_2=890.9kJ/kg`. The specific volume at
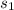`s_1` can then be determined as:

`\alpha_1=(RT_1)/(P_1)\\\\=(0.287* 300)/(95) m^3/kg\\0.906316m^3/kg`

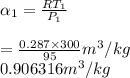
Specific volume,
`s_2`:

`\alpha_2=(\alpha_1)/(r)\\=(0.906316)/(16)m^3/kg\\=0.05664m^3/kg`

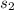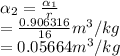
The pressures at
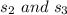`s_2 \ and\ s_3` is:

`P_2=P_3=(RT_2)/(\alpha_2)\\\\=(0.287*862)/(0.05664)\\=4367.06kPa`

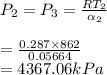
.The thermal efficiency=> maximum temperature at
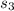`s_3` can be obtained from the expansion work at constant pressure during
`s_2-s_3`

`\bigtriangleup \omega_2_-_3=P(\alpha_3-\alpha_2)\\R(T_3-T_2)=P\alpha(r_c-1)\\T_3=T_2+(P\alpha_2)/(R)(r_c-1)\\\\=(862+(4367* 0.05664)/(0.287)(2-1))K\\=1723.84K`

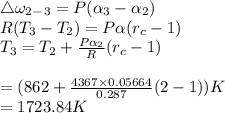
b.Relative SV and enthalpy at
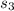`s_3` are obtained for the given temperature with interpolation with data from A-17 :
`a_r_3=4.553 \ and\ h_3=1909.62kJ/kg`

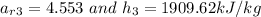
Relative SV at
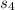`s_4` is

`a_r_4=(r)/(r_c)\alpha _r_3`

=
`=(16)/(2)*4.533\\=36.424`

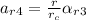
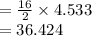
Thermal efficiency occurs when the heat loss is equal to the internal energy decrease and heat gain equal to enthalpy increase;

`n=1-(q_o)/(q_i)\\=1-(u_4-u_1)/(h_3-h_2)\\=1-(65903-214.07)/(1909.62-890.9)\\=0.563`

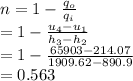
Hence, the thermal efficiency is 0.563

c. The mean relative pressure is calculated from its standard definition:

`MEP=(\omega)/(\alpa_1-\alpa_2)\\=(q_i-q_o)/(\alpha_1(1-1/r))\\=(1909.62-890.9-(65903-214.7))/(0.90632(1-1/16))\\=674.95kPa`

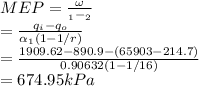
Hence, the mean effective relative pressure is 674.95kPa