Answer:
Explanation:
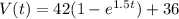 is given value in dollars of a stock in t months after it is purchased.
is given value in dollars of a stock in t months after it is purchased.
a) Substitute 1 for t
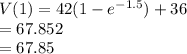
V(12) =
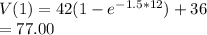
b) Find derivative for V

c) When V(t) = 75
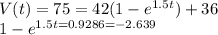
d) As t tends to infinity, exponent being in negative t tends to 0
So V tends to 42(1-0)+36 = 78