Question

Iodine-131 is a beta emitter used as a tracer in radio immunoassays in biological systems. It follows first order kinetics. The half-life if iodine-131 is 8.04 days. If you start with 8.0 grams of iodine-131, how many grams would remain at 39 days

Answer 1

Answer: The amount of Iodine-131 remain after 39 days is 0.278 grams

Step-by-step explanation:

The equation used to calculate rate constant from given half life for first order kinetics:

`t_(1/2)=(0.693)/(k)`

where,

`t_(1/2)` = half life of the reaction = 8.04 days

Putting values in above equation, we get:

`k=(0.693)/(8.04days)=0.0862days^(-1)`

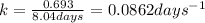
Rate law expression for first order kinetics is given by the equation:

`k=(2.303)/(t)\log([A_o])/([A])`

where,

k = rate constant =
`0.0862days^(-1)`

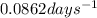
t = time taken for decay process = 39 days

`[A_o]` = initial amount of the sample = 8.0 grams

[A] = amount left after decay process = ?

Putting values in above equation, we get:

`0.0862=(2.303)/(39)\log(8.0)/([A])`

`[A]=0.278g`

Hence, the amount of Iodine-131 remain after 39 days is 0.278 grams