Question

In a study of government financial aid for college​ students, it becomes necessary to estimate the percentage of​ full-time college students who earn a​ bachelor's degree in four years or less. Find the sample size needed to estimate that percentage. Use a 0.02 margin of error and use a confidence level of 99​%. Complete parts​ (a) through​ (c) below.

a. Assume that nothing is known about the percentage to be estimated.n = ________b. Assume prior studies have shown that about 55% of​ full-time students earn​ bachelor's degrees in four years or less.n = _______c. Does the added knowledge in part​ (b) have much of an effect on the sample​ size?

Answer 1

(a) The sample size required is 2401.

(b) The sample size required is 2377.

(c) Yes, on increasing the proportion value the sample size decreased.

Explanation:

The confidence interval for population proportion p is:

`CI=\hat p\pm z_(\alpha/2)\sqrt{(\hatp(1-\hat p))/(n)}`

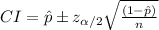
The margin of error in this interval is:

`MOE=z_(\alpha/2)\sqrt{(\hatp(1-\hat p))/(n)}`

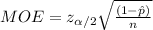
The information provided is:

MOE = 0.02

`z_(\alpha/2)=z_(0.05/2)=z_(0.025)=1.96`

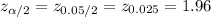
(a)

Assume that the proportion value is 0.50.

Compute the value of n as follows:

`MOE=z_(\alpha/2)\sqrt{(\hat p(1-\hat p))/(n)}\\0.02=1.96* \sqrt{(0.50(1-0.50))/(n)}\\n=(1.96^(2)*0.50(1-0.50))/(0.02^(2))\\=2401`

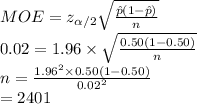
Thus, the sample size required is 2401.

(b)

Given that the proportion value is 0.55.

Compute the value of n as follows:

`MOE=z_(\alpha/2)\sqrt{(\hat p(1-\hat p))/(n)}\\0.02=1.96* \sqrt{(0.55(1-0.55))/(n)}\\n=(1.96^(2)*0.55(1-0.55))/(0.02^(2))\\=2376.99\\\approx2377`

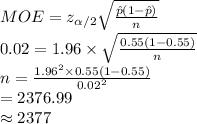
Thus, the sample size required is 2377.

(c)

On increasing the proportion value the sample size decreased.