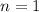2 Answers

Hugo Nava Kopp · Answer 1 · 2021-05-23T15:37:51+0000

For this case we have the following expression:

$\frac {216 ^ {n-2}} {(\frac {1} {36}) ^ {3n}} = 216$

We multiply both sides by:
$(\frac {1} {36}) ^ {3n}$

$216 ^ {n-2} = 216 * (\frac {1} {36}) ^ {3n}$

We divide both sides by 216:

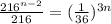
$\frac {216 ^ {n-2}} {216} = (\frac {1} {36}) ^ {3n}$

To divide powers of the same base, we place the same base and subtract the exponents:

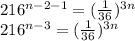
$216 ^ {n-2-1} = (\frac {1} {36}) ^ {3n}\\216 ^ {n-3} = (\frac {1} {36}) ^ {3n}$

Rewriting:

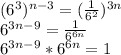
$(6 ^ 3) ^ {n-3} = (\frac {1} {6 ^ 2}) ^ {3n}\\6 ^ {3n-9} = \frac {1} {6 ^ {6n}}\\6^( 3n-9) * 6^( 6n) = 1$

To multiply powers of the same base, we place the same base and add the exponents:

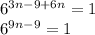
$6^( 3n-9 + 6n) = 1\\6^( 9n-9) = 1$

We know that any number raised to zero is 1,
a ^ 0 = 1.

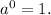
So, for equality to be true:

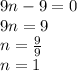
$9n-9 = 0\\9n = 9\\n = \frac {9} {9}\\n = 1$

Answer:

n = 1

Please log in or register to add a comment.