Question

Lauren coordinates a construction projects for a cement company. A government project requires constructing two rectangular concrete slabs of dimensions 24× 24× 1 feet. Additionally, the company sends a 20% surplus of concrete to ensure the job can be completed. If a cement truck can carry a maximum of 8 cubic yards of cement, what's the fewest number of trucks that Lauren should send? A)1 B)2 C)3 D)4 E)5lar

Answer 1

The fewest number of trucks Lauren should send is D) 4 trucks.

Explanation:

Step 1:

The rectangular slab's dimensions are
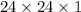`24 * 24 * 1` feet. Each truck can carry 8 cubic yards of cement.

First, we need to determine the volume of the slabs in yards. 1 foot = 0.333 yards. So 24 feet =
`24* 0.3333` = 8 yards.

The volume of the slab =
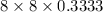`8 * 8 * 0.3333` = 21.3312 cubic yards.

Step 2:

The company sends a surplus of 20% to make sure the job can be completed. So the total cement sent is the required volume and an extra 20%.

The total cement sent = The required cement + 20%.

= 21.3312 + 20% = 25.597 cubic yards.

Step 3:

So to find the number of trucks needed, we divide the cement sent by the load each truck can carry. Each truck can carry 8 cubic yards of cement. So

The number of trucks needed =
`(therequiredload)/(load per truck) = (25.597)/(8) = 3.199625.`

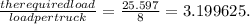
If 3.199 trucks are needed, it means 4 trucks are needed which is option D.