Question

You stand 17.5 m from a wall holding a tennis ball. You throw the tennis ball at the wall at an angle of 22.5∘ from the ground with an initial speed of 24.5 m/s. At what height above its initial position does the tennis ball hit the wall? Ignore any effects of air resistance.

Answer 1

The height of the ball would be 4.32 m

Step-by-step explanation:

Given-

Distance from the ball, s = 17.5 m

Angle of projection, θ = 22.5°

Initial speed, u = 24.5 m/s

Height, h = ?

Let t be the time taken.

Horizontal speed,
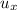`u_(x)` = u cosθ

= 24.5 * cos 22.5°

= 24.5 * 0.924

= 22.64 m/s

Vertical velocity,
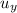`u_(y)` = u sinθ

= 24.5 * sin 22.5°

= 24.5 * 0.383

= 9.38 m/s

We know,

`x = u * cos (theta) * t`

`17.5 = 22.64 * t\\\\t = 0.77s`

To calculate the height:

`h = ut - (1)/(2)gt^2`

`h = u sin (theta)t - (1)/(2) gt^2`

`h = 9.38 * 0.77 - (1)/(2) * 9.8 * (0.77)^2\\ \\h = 7.22 - 2.90\\\\h = 4.32m\\`

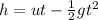
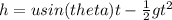
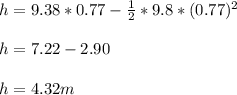
Therefore, height of the ball would be 4.32 m