Question

Statuary Hall is an elliptical room in the United States Capitol in Washington, D.C. The room is also called the Whispering Gallery because a person standing at one focus of the room can hear even a whisper spoken by a person standing at the other focus. The dimensions of Statuary Hall are 46 feet wide by 97 feet long. A) Find an equation that models the shape of the room.B) How far apart are the two foci?C) What is the area of the floor of the room?

Answer 1

Answer: a)
`(x^(2) )/(2352.25 )` +
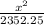`(y^(2) )/(529)` = 1

b) The distance of two foci is 85.4 feet

c) Area = 3502.67 square feet

Explanation: a) An ellipse has the equation in the form of:

`(x^(2) )/(a^(2) )`+
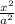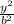`(y^(2) )/(b^(2) )` = 1, where a is the horizontal axis and b is the vertical axis.

For the Statuary Hall, a =
`(97)/(2)` = 48.5 and b =
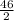`(46)/(2)` = 23, so the equation will be

`(x^(2) )/(2352.25 )` +
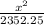`(y^(2) )/(529)` = 1.

b) To determine the distance of the foci, we have to calculate 2c, where c is the distance between one focus and the center of the ellipse. To find c, as a, b and c create a triangle with a as hypotenuse:

`a^(2)` =
`b^(2) + c^(2)`

`c^(2) = a^(2) - b^(2)`

c =
`\sqrt{48.5^(2) - 23^(2) }`

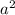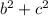
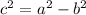
c = 42.7

The distance is 2c, so 2·42.7 = 85.4 feet.

The two foci are 85.4 feet apart.

c)The area of an ellipse is given by:

A = a.b.π

A = 48.5 · 23 · 3.14

A = 3502.67 ft²

The area of the floor room is 3502.67ft².