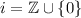Question

The equation r(t)= (3t+9)i+(sqrt(2)t)j+(t^2)k is the position of a particle in space at time t. Find the angle between the velocity and acceleration vectors at time t=0

What is the angle? ______radians

Answer 1

`\theta= (\pi)/(2) +\pi \cdot i`, for all
`i = \mathbb{Z} \cup\{0\}`

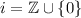
Explanation:

The velocity vector is found by deriving the position vector depending on the time:

`\dot r(t)= v (t) = 3 \cdot i +√(2) \cdot j + 2\cdot t \cdot k`

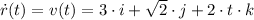
In turn, acceleration vector is found by deriving the velocity vector depending on time:

`\ddot r(t) = \dot v(t) = a(t) = 2 \cdot k`

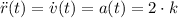
Velocity and acceleration vectors at
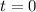`t = 0` are:

`v(0) = 3\cdot i + √(2) \cdot j\\a(0) = 2 \cdot k\\`

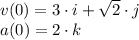
Norms of both vectors are, respectively:

`||v(0)||\approx 3.317\\||a(0)|| \approx 2`

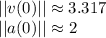
The angle between both vectors is determined by using the following characteristic of a Dot Product:

`\theta = \cos^(-1)((v(0) \bullet a(0))/(||v(0)||\cdot ||a(0)||))`

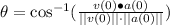
Given that cosine has a periodicity of
`\pi`. There is a family of solutions with the form:

`\theta= (\pi)/(2) +\pi \cdot i`, for all
`i = \mathbb{Z} \cup\{0\}`