Answer:
a)
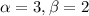
b) 0.0620
Explanation:
We are given the following in the question:
Population mean,
 = 6
= 6
Variance,
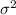 = 12
= 12
a) Value of
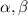
We know that
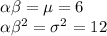
Dividing the two equations, we get,
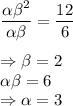
b) probability that on any given day the daily power consumption will exceed 12 million kilowatt hours.
We can write the probability density function as:
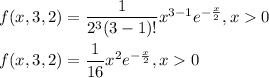
We have to evaluate:
![P(x >12)\\\\= (1)/(16)\displaystyle\int^(\infty)_(12)f(x)dx\\\\=(1)/(16)\bigg[-2x^2e^{-(x)/(2)}-2\displaystyle\int xe^{-(x)/(2)}dx}\bigg]^(\infty)_(12)\\\\=(1)/(8)\bigg[x^2e^{-(x)/(2)}+4xe^{-(x)/(2)}+8e^{-(x)/(2)}\bigg]^(\infty)_(12)\\\\=(1)/(8)\bigg[(\infty)^2e^{-(\infty)/(2)}+4(\infty)e^{-(\infty)/(2)}+8e^{-(\infty)/(2)} -( (12)^2e^{-(12)/(2)}+4(12)e^{-(12)/(2)}+8e^{-(12)/(2)})\bigg]\\\\=0.0620](https://img.qammunity.org/2021/formulas/mathematics/college/4x52dy61hn0384t29dgohqocipgfj30w2y.png)
0.0620 is the required probability that on any given day the daily power consumption will exceed 12 million kilowatt hours.