Question

Let ​f(x)equals

7xplus4
and ​g(x)equals
4xminus5.
Find ​(fplus
​g)(x),
​(fminus
​g)(x),
​(fg)(x), and left parenthesis StartFraction f Over g EndFraction right parenthesis
​(x).
Give the domain of each.

Answer 1

Explanation:

We have , f(x) = 7x + 4 & g(x) = 4x-5.

A: ( f + g )( x )

We know that sum of two functions is as simple addition so,

`( f + g ) (x) = f(x) + g(x)\\( f + g ) (x) = 7x+4 + 4x-5\\( f + g ) (x) = 11x - 1`

B: ( f - g ) ( x )

We know that difference of two functions is as simple subtraction so,

`( f - g )(x) = f(x) - g(x)\\( f - g )(x) = 7x + 4 - ( 4x - 5 )\\( f - g )(x) = 3x + 9`

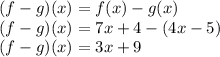
C:( fg ) (x)

We know that multiply of two functions is as simple multiplication so,

`( fg) (x)= f(x).g(x)\\( fg) (x) = (7x + 4)(4x-5)\\( fg) (x) = 28x^(2) - 35x + 16x - 20\\( fg) (x) = 28x^(2) -19x -20`

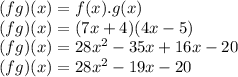
D: (f/g)(x)

We know that Division of two functions is as simple division so,

`((f)/(g))(x) = (f(x))/(g(x)) \\((f)/(g))(x) = (7x +4)/(4x-5)`

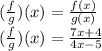
Since, both f(x) and g(x) are real valued functions , ∴ Domain of both are real numbers.