Step-by-step explanation:
As it is given that,
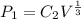
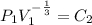
As the system is not gaining or losing heat. So, it is an adiabatic process in an assumed ideal gas. The polytropic extent n is
 .
.
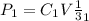
=
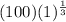
= 100 kpa

=

= 158.74 kpa
Now, work done by the air is as follows.
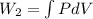
=
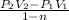
=
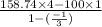
= 401.22 kJ
Work done by the air is as follows.
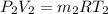
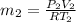
=

= 7.424 kg
Thus, we can conclude that final mass of air is 7.424 kg and work done by the air is 401.22 kJ.