Question

Antimony pentachloride decomposes according to this equation: SbCl5(g)⇌SbCl3(g)+Cl2(g) An equilibrium mixture in a 5.00-L flask at 448 °C contains 3.85 g of SbCl5, 9.14 g of SbCl3, and 2.84 g of Cl2. How many grams of each will be found if the mixture is transferred into a 2.00-L flask at the same temperature?

Answer 1

The mass of SbCl₅ in 2.00-L flask = 14.95 g

The mass of SbCl₃ in 2.00-L flask = 11.41 g

The mass of Cl₂ in 2.00-L flask = 3.55 g

Step-by-step explanation:

Equation for the reaction is given as:

SbCl5(g)⇌SbCl3(g)+Cl2(g)

Equilibrium constant
`K__C` =
`([SbCl_3][Cl_2])/([SbCl_5])`

Let's calculate their respective number of moles; followed by their molar concentrations.

Since number of moles
`(n_x)` =
`(mass)/(molarmass)`

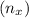Therefore;

For SbCl₅(g)

mass given =3.85 g

molar mass = 299 g/mol

number of moles of SbCl₅(g) will be:

`n__((SbCL_5))` =
`(3.85)/(299)`

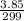
`n__((SbCL_5))` = 0.0128 moles

For SbCl₃(g);

mass given = 9.14 g

molar mass = 228.13 g/mol

number of moles of SbCl₃(g) will be:

`n__((SbCL_3))` =
`(9.14g)/(228.13)`

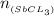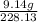
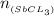`n__((SbCL_3))` = 0.0400 moles

For Cl₂(g)

mass given 2.84 g

molar mass = 70.906 g/mol

number of moles of Cl₂(g) will be:

`n__{(Cl_2)` =
`(2.84)/(70.906)`

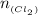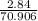
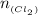`n__{(Cl_2)` = 0.0400 moles

Molar Concentration =
`(number of mole)/(volume)`

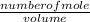
Their respective molar concentration can be calculated as follows:

Molar Concentration for SbCl₅(g)

[SbCl₅(g)] =
`(0.0128)/(5.00)`

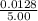
= 0.00256

= 2.56 × 10⁻³ M

Molar Concentration for SbCl₃(g)

[SbCl₃(g)] =
`(0.0400)/(5.00)`

= 0.008

= 8 × 10⁻³ M

Molar Concentration for Cl₂(g)

[Cl₂(g)] =
`(0.0400)/(5.00)`

= 0.008

= 8 × 10⁻³ M

Again, we knew our Equilibrium constant
`K__C` =
`([SbCl_3][Cl_2])/([SbCl_5])`

∴

`K__C` =
`([8*10^(-3)][8*10^(-3)])/([2.56*10^(-3)])`

`K__C` = 0.025

For the equilibrium mixture in 5.00 L flask at 448°C;
`K__C` = 0.025

∴ For 2.00 L;

Let (y) M be the concentration of SbCl₅(g), SbCl₃(g) and Cl₂(g)

∴

`K__C` =
`([y][y])/([y])`

`K__C` = y

0.0025 = y

∴ For SbCl₅(g), SbCl₃(g) and Cl₂(g) in 2.00-L; their respective Molar Concentration is 0.025 M each.

So, since numbers of moles =
`(mass)/(molarmass)`

mass = numbers of moles × molar mass

Also, numbers of moles = Molar Concentration × Volume

∴ mass = Molar Concentration × Volume × molar mass

For SbCl₅(g);

mass = 0.025 × 2 × 229g

= 14.95 g

For SbCl₃(g);

mass = 0.025 × 2 × 228.13

= 11.4065 g

≅ 11.41 g

For Cl₂(g):

mass = 0.025 × 2 × 70.906

= 3.5453 g

≅ 3.55 g

∴

The mass of SbCl₅ in 2.00-L flask = 14.95 g

The mass of SbCl₃ in 2.00-L flask = 11.41 g

The mass of Cl₂ in 2.00-L flask = 3.55 g