Question

Consider the reaction 3X + 2Y → 5C + 4D With excess Y, how many moles of X are needed to produce 21.00 moles of D?​

Answer 1

`\huge \tt༆ Answer ༄`

According to given equation,

`{ \qquad{ \sf{ \dashrightarrow}}}  \:  \: \sf \:3 \: moles \: X \rightarrow \: 4 \: moles \: D`

Now, let's use simple unitary method ~

`{ \qquad{ \sf{ \dashrightarrow}}}  \:  \: \sf \: (3)/(4) \: moles \: X \rightarrow \: 1 \: moles \: D`

`{ \qquad{ \sf{ \dashrightarrow}}}  \:  \: \sf \: (3)/(4) * 21 \: moles \: X \rightarrow 21 \: moles \: D`

`{ \qquad{ \sf{ \dashrightarrow}}}  \:  \: \sf \:15.75 \: moles \: X \rightarrow 21 \: moles \: D`

Therefore, request moles of X is 15.75 moles !

Answer 2

`\huge\boxed{\sf 15.75\ moles }`

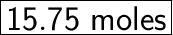
Step-by-step explanation:

Given reaction is:

3X + 2Y → 5C + 4D

In this reaction, we have:

For 3 moles of X, 4 moles of D

So,

4D = 3X

Divide both sides by 4

D = 3X/4

Multiply both sides by 21

21D = 3X/4 × 21

21D = 3*21X/4

21D = 63X/4

21D = 15.75X

So, to produce 21 moles of D, we need 15.75 moles of X.

`\rule[225]{225}{2}`

Hope this helped!

~AH1807