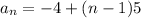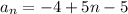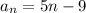Answer:
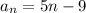
Explanation:
we have the following sequence,

where
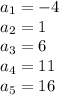
so
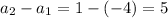 ----->
----->
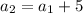
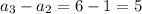 ----->
----->
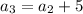
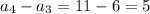 ----->
----->
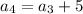
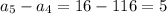 ----->
----->
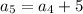
Note there is a common difference d=5 between consecutive terms
This indicates the sequence is arithmetic with n th term
so
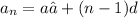
where a₁ is the first term and d the common difference
substitute