Question

A volcanic eruption throws a boulder that lands 1.00 km horizontally from the crater. If the volcanic rocks were launched at an angle of 40° with respect to the horizontal and landed 900 m below the crater, (a) what would be boulders initial velocity and (b) what is the time of flight?

Answer 1

a) v₀ = 69.29 m / s , b) t = 18.84 s

Step-by-step explanation:

a) For this exercise we will use the projectile launch equations

x = v₀ₓ t

y = y₀ +
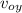`v_(oy)` t - ½ g t²

Let's fix our reference system on the volcano, so the horizontal distance x = 1 km = 1000 m and the vertical distance y = -900 m, the initial height of the crater is I = 0 m. Let's replace to find the speeds

v_{oy} = v₀ sin θ

v₀ₓ = v₀ cos θ

y = v₀ sin θ (x / v₀ cos θ) - ½ g (x / v₀ cos θ)2

y = x tan θ - ½ g x² / v₀² sec² θ

½ g x² sec² θ / v₀² = x tan θ - y

v₀² = ½ g x² sec² θ / (x tan θ –y)

Let's calculate

v₀² = ½ 9.8 1000² sec² 40 / (1000 tan 40 - (-900))

v₀ = √ (8.35 10⁶ / 1,739 10³)

v₀ = 69.29 m / s

b) Flight time

x = v₀ₓ t

t = x / v₀ cos θ

t = 1000 / 69.29 cos 40

t = 18.84 s