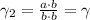Answer:
Explanation:
The question to be solved is the following :
Suppose that a and b are any n-vectors. Show that we can always find a scalar γ so that (a − γb) ⊥ b, and that γ is unique if
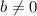 . Recall that given two vectors a,b a⊥ b if and only if
. Recall that given two vectors a,b a⊥ b if and only if
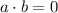 where
where
 is the dot product defined in
is the dot product defined in
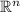 . Suposse that
. Suposse that
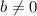 . We want to find γ such that
. We want to find γ such that
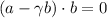 . Given that the dot product can be distributed and that it is linear, the following equation is obtained
. Given that the dot product can be distributed and that it is linear, the following equation is obtained

Recall that
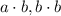 are both real numbers, so by solving the value of γ, we get that
are both real numbers, so by solving the value of γ, we get that
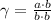
By construction, this γ is unique if
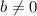 , since if there was a
, since if there was a
 such that
such that
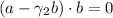 , then
, then