Answer:
each of the planets moving = 408.35 m/s
Step-by-step explanation:
given data
mass =
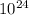 kg
kg
distance between two planets = 2 ×
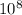 m
m
solution
if distance between two planet is 2 ×
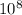 m it mean it's diameter so
m it mean it's diameter so
that radius will be 1 ×
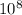 m
m
and
F =
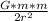 ................1
................1
and
F =
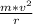 ..............2
..............2
so from equation 1 and 2 will be
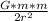 =
=
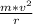
v =
 ............2
............2
put here value
v =

v = 408.35 m/s