Answer:
Proportion of all bearings falls in the acceptable range = 0.9973 or 99.73% .
Explanation:
We are given that the diameters have a normal distribution with a mean of 1.3 centimeters (cm) and a standard deviation of 0.01 cm i.e.;
Mean,
 = 1.3 cm and Standard deviation,
= 1.3 cm and Standard deviation,
 = 0.01 cm
= 0.01 cm
Also, since distribution is normal;
Z =
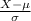 ~ N(0,1)
~ N(0,1)
Let X = range of diameters
So, P(1.27 < X < 1.33) = P(X < 1.33) - P(X <=1.27)
P(X < 1.33) = P(
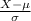 <
<
 ) = P(Z < 3) = 0.99865
) = P(Z < 3) = 0.99865
P(X <= 1.27) = P(
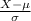 <
<
 ) = P(Z < -3) = 1 - P(Z < 3) = 1 - 0.99865
) = P(Z < -3) = 1 - P(Z < 3) = 1 - 0.99865
= 0.00135
P(1.27 < X < 1.33) = 0.99865 - 0.00135 = 0.9973 .
Therefore, proportion of all bearings that falls in this acceptable range is 99.73% .