Question # 1
Answer:
Therefore, the vertex form is:

And the vertex of the function is: (-2, -79)
Explanation:
Given the function

Factoring 15

Adding and subtracting the square of half the coefficient of
 .
.
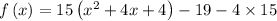
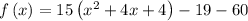
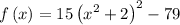
Therefore, the vertex form is:

And the vertex of the function is: (-2, -79)
Question # 2
Answer:
 is the vertex form of
is the vertex form of
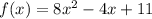 .
.
Explanation:
Given the function
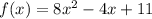
Factoring 8 from the first two terms
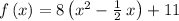
Next adding and subtracting the square of half the coefficient of the linear term
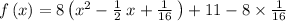

Factoring the perfect square trinomial
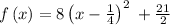
Therefore,
 is the vertex form of
is the vertex form of
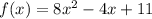 .
.