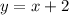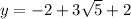Question

A positive real number is 2 less than another. When 4 times the larger is added to the square of the smaller, the result is 49. Find the numbers

Answer 1

The numbers are

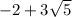`-2+3√(5)` and
`3√(5)`

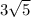
Explanation:

Let

x -----> the smaller positive real number

y -----> the larger positive real number

we know that

A positive real number is 2 less than another

so

`x=y-2`

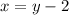
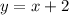`y=x+2` ----> equation A

When 4 times the larger is added to the square of the smaller, the result is 49

so

`4y+x^2=49` ----> equation B

substitute equation A in equation B

`4(x+2)+x^2=49`

solve for x

`x^2+4x-41=0`

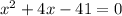
The formula to solve a quadratic equation of the form

`ax^(2) +bx+c=0`

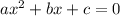
is equal to

`x=\frac{-b\pm\sqrt{b^(2)-4ac}} {2a}`

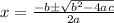
in this problem we have

`x^2+4x-41=0`

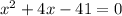
so

`a=1\\b=4\\c=-41`

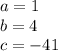
substitute in the formula

`x=\frac{-4\pm\sqrt{4^(2)-4(1)(-41)}} {2(1)}`

`x=\frac{-4\pm√(180)} {2}`

`x=\frac{-4\pm6√(5)} {2}`

`x=-2\pm3√(5)`

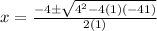
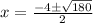
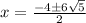
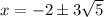
so

The positive real number is

`x=-2+3√(5)`

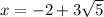
Find the value of y

`y=x+2`

`y=-2+3√(5)+2`

`y=3√(5)`