By law, sales tax is calculated on the discounted price of the shirts. Would the total cost of the shirts including the 6.5% sales tax be greater if the tax was applied before a 20% discount is taken, rather than after a 20% discount is taken?
Answer:
They will be the same
Explanation:
Sales tax applied before
Total costs before discount = (2*$ 10.98)+$ 11.50+(2*$ 15.99)=$ 65.44
Discount of 20% will be
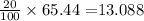
New price= 65.44-13.088=$52.352
Adding 6.5% sales tax we obtain
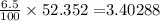
Total payment= 52.352+3.40288=55.75488
Sales Tax applied after
If 20% is taken after sales tax then the sales tax of 6.5 amounts to
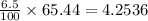
New price= 65.44+4.2536=$ 69.6936
Then 20% discount is
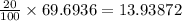
Discounted price after sales tax= 69.6936-13.93872
=55.75488
Both systems come to the same value hence there's no difference