Answer:
e. 0.0072
Explanation:
We are given that a bottling company uses a filling machine to fill plastic bottles with cola. And the contents vary according to a Normal distribution with Mean, μ = 298 ml and Standard deviation, σ = 3 ml .
Let Z =
 ~ N(0,1) where, Xbar = mean contents of six randomly
~ N(0,1) where, Xbar = mean contents of six randomly
selected bottles
n = sample size i.e. 6
So, Probability that the mean contents of six randomly selected bottles is less than 295 ml is given by, P(Xbar < 295)
P(Xbar < 295) = P(
 <
<
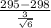 ) = P(Z < -2.45) = P(Z > 2.45)
) = P(Z < -2.45) = P(Z > 2.45)
Now, using z% score table we find that P(Z > 2.45) = 0.00715 ≈ 0.0072 .
Therefore, option e is correct .