Question

A surfboard shaper has to limit the cost of development and production to ​$ 215 per surfboard. He has already spent ​$ 30,240 on equipment for the boards. The development and production costs are ​$ 143 per board. The cost per board is 143x+30,240/x dollars. Determine the number of boards that must be sold to limit the final cost per board to $ 215.

Answer 1

420 boards.

Explanation:

We have been given the formula for cost per board as
`(143x+30240)/(x)`, where x represent number of boards. We are asked to find the number of boards that must be sold to limit the final cost per board to $215.

To find the number of boards, we will equate cost of x boards by 215 and solve for x as shown below:

`(143x+30240)/(x)=215`

`(143x+30240)/(x)*x=215*x`

`143x+30240=215x`

`143x-143x+30240=215x-143x`

`30240=72x`

`72x=30240`

`(72x)/(72)=(30240)/(72)`

`x=420`

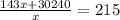
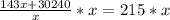
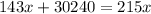
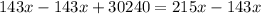
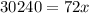
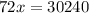
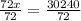
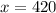
Therefore, 420 boards must be sold to limit the final cost per board to $215.