Answer:
![\displaystyle \textcolor{black}{4.}\:[-3, -5]](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/h1hy8e29b46lq5y5hrdx.png)
![\displaystyle \textcolor{black}{3.}\:[8, -1]](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/5kl3ud7m9jwml22fq2uf.png)
![\displaystyle \textcolor{black}{2.}\:[4, -7]](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/sn0r6e7sgutwwkuvk2ln.png)
![\displaystyle \textcolor{black}{1.}\:[3, -2]](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/qgfiyhsqb91ivdlkhrgk.png)
Explanation:
When using the Elimination method, you eradicate one pair of variables so they are set to zero. It does not matter which pair is selected:
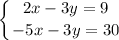
{2x - 3y = 9
{⅖[−5x - 3y = 30]

------------------------------------------------------------------------------------------
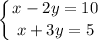
{x - 2y = 10
{⅔[x + 3y = 5]
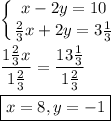
_______________________________________________
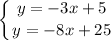
{y = −3x + 5
{−⅜[y = −8x + 25]
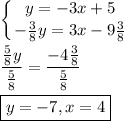
------------------------------------------------------------------------------------------
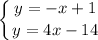
{y = −x + 1
{¼[y = 4x - 14]

_______________________________________________
I am joyous to assist you at any time.