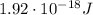Question

What is the electric potential energy of an electron at the negative end of the cable, relative to the positive end of the cable? In other words, assume that the electric potential of the positive terminal is 0 VV and that of the negative terminal is −12V−12V. Recall that e=1.60×10−19Ce=1.60×10−19C

Answer 1

Electric potential energy at the negative terminal:
`1.92\cdot 10^(-18)J`

Step-by-step explanation:

When a particle with charge
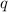`q` travels across a potential difference
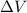`\Delta V`, then its change in electric potential energy is

`\Delta U = q \Delta V`

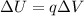
In this problem, we know that:

The particle is an electron, so its charge is

`q=-1.60\cdot 10^(-19)C`

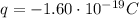
We also know that the positive terminal is at potential

`V_+=0V`

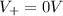
While the negative terminal is at potential

`V_-=-12 V`

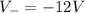
Therefore, the potential difference (final minus initial) is

`\Delta V = -12-0 = -12 V`

So, the change in potential energy of the electron is

`\Delta U = (-1.6\cdot 10^(-19))(-12)=1.92\cdot 10^(-18)J`

This means that the electron when it is at the negative terminal has
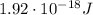`1.92\cdot 10^(-18)J` of energy more than when it is at the positive terminal.

Since the potential at the positive terminal is 0, this means that the electric potential energy of the electron at the negative end is

`1.92\cdot 10^(-18)J`