Question

An amusement park ride consists of a car moving in a vertical circle on the end of a rigid boom of negligible mass. The combined weight of the car and riders is 5.30 kN, and the radius of the circle is 12.0 m. At the top of the circle, (a) what is the force FB on the car from the boom (using the minus sign for downward direction) if the car's speed is v = 3.60 m/s? (b) What is FB if v = 14.0 m/s? Use g=9.80 m/s2.

Answer 1

Step-by-step explanation:

As the force is given as 5.30 kN or
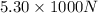`5.30 * 1000 N`. Hence, mass will be calculated as follows.

`F_(w)` = mg

`5.30 * 10^(3) = m * 9.8 m/s^(2)`

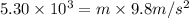
m = 540.816 kg

(a) At the top, centripetal force
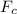`F_(c)` is acting upwards and the weight of the riders and car,
`F_(w)` will be acting downwards.

Therefore, force on the car by the boom will be calculated as follows.

`F_(B) = F_(w) - F_(c)`

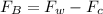
or,
`F_(B) = mg - (mv^(2))/(r)`

=
`5.30 * 1000 N - (540.816 kg * (3.60)^(2))/(12)`

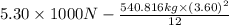
= 4715.919 N

Hence, the force
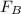`F_(B)` on the car from the boom is 4715.919 N. This means that the car will be hanging on the boom and the boom will exert an upward force.

(b) Now at the top, centripetal force
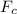`F_(c)` will be acting upwards and the weight of cars and car riders will be acting in the downwards direction.

Hence, we will calculate the force on car by the boom as follows.

`F_(B) = F_(w) - F_(c)`

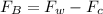
or,
`F_(B) = mg - (mv^(2))/(r)`

=
`5.30 * 1000 N - (540.816 kg * (14.0)^(2))/(12)`

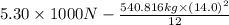
= -3533.33 N

Therefore, car will be pushing on the boom and the boom will exert a downward force.