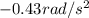To solve this problem we will apply the concepts related to the cinematic equations of angular motion. On these equations, angular acceleration is defined as the squared difference of angular velocity over twice the radial displacement. This is mathematically:
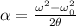
Our values are,
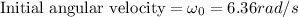
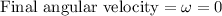
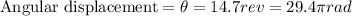
Replacing,
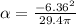

Therefore the angular acceleration is