Question

Two dice are tossed. Assume that each possible outcome has a
`(1)/(36)` probability. Let A be the event that the sum of the faces showing is 6, and let B be the event that the face showing on one die is twice the face showing on the other. Calculate
`P(A \cap B^C)`.

Answer 1

`P(A n B^(c) ) = (1)/(12)`

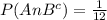
Explanation:

Total possible outcome is:

`\left[\begin{array}{cccccc}1,1&1,2&1,3&1,4&1,5&1,6\\2,1&2,2&2,3&2,4&2,5&2,6\\3,1&3,2&3,3&3,4&3,5&3,6\\4,1&4,2&4,3&4,4&4,5&4,6\\5,1&5,2&5,3&5,4&5,5&5,6\\6,1&6,2&6,3&6,4&6,5&6,6\end{array}\right]` = 36 total possible outcome

Event A(sum of the faces showing 6) = (1, 5), (2, 4), (3, 3), (4, 2), (5, 1) = 5

Event B(one die twice the face of another) = (1, 2), (2, 1), (2, 4), (3, 6), (4, 2), (6, 3)

B' (B-complement) comprises of all the other possible outcome except those listed for B.

Therefore, among all the other possible outcome, those possible together with Event A are {(1, 5), (3, 3), (5, 1)} = 3

`P(A n B^(c) ) = (3)/(36) = (1)/(12)`

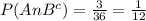
1/12 is the probability of events that can occur in Event A and the complement of B.