Question

please answer this question fast and please show all your work because I am locking for how can you do it please ​

Answer 1

Option C is the correct values of the relationship between the number of cakes the baker makes and the number of bags of flour uses.

Solution:

Option A: Ratio of cakes baked to bags of flour used

`$(9)/(4), (18)/(6)=(3)/(1) \text { and } (27)/(9)=(3)/(1)`

Here the ratios are not same.

So, this option is not true.

Option B: Ratio of cakes baked to bags of flour used

`$(6)/(2)=(3)/(1), (12)/(4)=(3)/(1) \text { and } (18)/(6)=(3)/(1)`

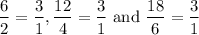
Here the ratios are same.

So, this option is true.

Option C: Ratio of cakes baked to bags of flour used

`$(4)/(9), (6)/(18)=(1)/(3) \text { and } (9)/(17)`

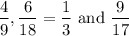
Here the ratios are not same.

So, this option is not true.

Option D: In this table cakes baked is 6 and the bags of flour is 18.

But a baker made 18 cakes using 6 bags of flour.

So, this option is not true.

Hence option C is the correct values of relationship between the number of cakes the baker makes and the number of bags of flour uses.