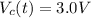Question

Consider that the capacitor is initially not charged and the voltage of the battery is 3.00 V. The switch is closed at ???????? = 0. Using the appropriate given formulas, write down below the voltage ????????cc(????????) on the capacitor and the current ????????(????????) in the circuit.

. For the R-C circuit shown in Figure 1 on Page 3, ???????? = 100 ???????? and ???????? = 1.00 ????????. What is the time constant of the R-C circuit?

Consider that the capacitor is initially fully charged to 3.00 V. The switch is opened at ???????? = 0. Using the appropriate given formulas, write down below the voltage ????????cc(????????) on the capacitor and the current ????????(????????) in the circuit.

Answer 1

• Your question has some difficulties in symbols and variables to read. That's why I am re-writing (re-arranged) the Question's statement again which is:

Question:

For the R-C circuit shown in figure 1 on page 3, R= 100 ohms and C=1.00F.

A)What is the time constant of the R-C circuit?

B) consider that the capacitor is initially not charged and the voltage of the battery is 3.00 V. The switch is closed at t=0. Using the appropriate formulas, write down the voltage Vc(t) on the capacitor and the current I(t) in the circuit.

C) consider that the capacitor is initially fully charged to 3.00 V. The switch is open at t=0. Using the appropriate formulas, write down the voltage Vc(t) on the capacitor and current I(t) in the circuit.

• Your Question is missing the figure as well, which is attached below, please find it in the attached file.

Answer:

Part.A

100 seconds

Part.B

Vc(t)=0V

I(t)=30mA

Part.C

Vc(t)=3.0V

I(t)=0A

Step-by-step explanation:

Given Data:

Resistance=R=100 ohms

Capacitance=C=100F

Part.A: Time constant=T=?

Time constant:

Time required to charge the capacitor upto 63.23% of the applied voltage is called a Time constant.

As we know that time constant for the RC (circuit having only resistor and capacitor) is equal to product of resistance and capacitance

`T=R*C`

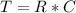
so
`T=100*1`

`T=100seconds`

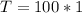
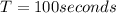
Part.B: Vc(t) and I(t)=? If switch is closed

Given Data:

Voltage of the battery=Vs=3.0V

Capacitor is not charged initially and switch is closed at t=0 means that the circuit will become short (because capacitor is charging) in which current I(t) exist and voltage Vc(t) is zero.

Short circuit:

The circuit in which current is maximum and voltage is zero.

Thus voltage on the capacitor is Vc(t)=0V

Now we can find I(t) as follow:

Thus, Applying Kirchoff's Law (sum of all voltages is zero) on the circuit in the figure attached below, we get

`V_(s)-V_(R)-V_(c)(t) =0`

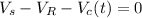
putting values, we get

`3.0-I(t)*R-0=0`

`I(t)*100=3`

`I(t)=(3)/(100)`

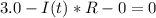
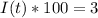
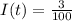
Current in the circuit is
`I(t)=0.03A\ OR\ 30mA`

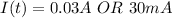
Part.c: Vc(t) and I(t)=? if switch is opened

If switch is opened and capacitor is fully charged then the capacitor will act as a battery and will supply will voltage as it has stored the charge. The switch is open it means the circuit is open.

So current I(t) in the circuit is zero=0A

and voltage Vc(t) exist which can be found as:

Applying Kirchoff's law, we get

`V_(s) -V_(c)(t)=0 \\V_(R)\ does\ not\ exist\ because\ current\ in\ the\ circuit\ or\ through\ resistor\ is\ zero`

`V_(s)=V_(c)(t)`

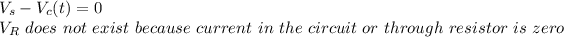
thus voltage on the capacitor is
`V_(c)(t)=3.0V`