Question

Scando-Germanic friend, Odd Zahlen, often brings a die to class to answer multiple-choice final exam questions. Each multiple-choice question on this particular examination consists of three choices, and Odd decides to pick answer (a) if a 1 or 2 appears on a roll of the die, to pick (b) if a 3 or 4 appears on the die, or to pick (c) if a 5 or 6 appears. Assume that the correct answers are uniformly distributed among the choices (a), (b), and (c). What is the probability of obtaining exactly 5 correct answers on a ten question examination using this method?

Answer 1

The probability of obtaining exactly 5 correct answers on a ten question examination is 0.1366.

Explanation:

Each multiple-choice questions has three options (a), (b) and (c).

The probability of getting a correct answer is,
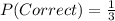`P(Correct)=(1)/(3)` since one of the three options is correct.

But this students has an unique way of selecting the answers.

He rolls a die and according to the result of the die he marks the answers.

The sample space of rolling a die is: S = {1, 2, 3, 4, 5, 6}

Odds of picking the three options are as follows:

• To pick (a): If 1 or 2 rolls of the die.

The probability to pick (a) is,

`P (Selecting\ (a))=(2)/(6)=(1)/(3)`

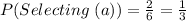
• To pick (b): If 3 or 4 rolls of a die.

The probability to pick (a) is,

`P (Selecting\ (b))=(2)/(6)=(1)/(3)`

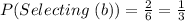
• To pick (c): If 5 or 6 rolls of the die.

The probability to pick (a) is,

`P (Selecting\ (c))=(2)/(6)=(1)/(3)`

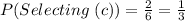
Thus, all the three options have the equal probability of being picked.

Let X = Number of correct answers.

The number of questions is, n = 10 and probability of selecting a correct option is , p =
`(1)/(3)`.

The random variable X follows Binomial distribution.

The probability function is:

`P(X = x)={n\choose x}p^(x)(1-p)^(n-x)`

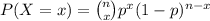
Compute the probability of obtaining exactly 5 correct answers on a ten question examination as:

`P(X = 5)={10\choose 5}((1)/(3) )^(5)(1-(1)/(3) )^(10-5)=0.1366`

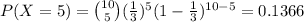
Thus, the probability of obtaining exactly 5 correct answers on a ten question examination is 0.1366.