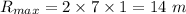Question

Trained dolphins are capable of a vertical leap of 7.0 m straight up from the surface of the water - an impressive feat. Suppose you could train a dolphin to launch itself out of the water at this same speed but at an angle. What maximum horizontal range could the dolphin achieve?

Answer 1

Answer:14 m

Step-by-step explanation:

Given

Vertical jump make by the dolphin is given by
`h=7\ m`

Suppose the dolphin jump with an initial velocity of
`u`

so u is given by
`u^2=2\cdot g\cdot h`

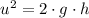
If dolphin launches at an angle
`\theta` then maximum horizontal range is given by

assuming the of Dolphin to be Projectile so range is given by

`R=(u^2\sin 2\theta )/(g)`

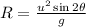
substitute the value of
`u^2`

`R=(2* 9.8* 7\sin 2\theta )/(9.8)`

`R=2h\sin 2\theta`

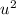
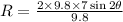
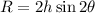
Range will be maximum for
`\theta =45^(\circ)`

thus
`R_(max)=2* 7* 1=14\ m`