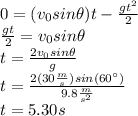Question

A student launches a small 0.5 kg rocket with an initial speed of 30 m/s at an angle of 60°. Approximately how much time will the rocket spend in the air? Air resistance is negligible.

Answer 1

`t=5.30s`

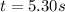
Step-by-step explanation:

The high reached by a proyectile in an uniformly accelerated motion is given by:

`y=v_(0y)t-(gt^2)/(2)`

The time that the rocket spends in the air is obtained for y = 0, since this is the time that the rocket travels before touching the ground. Recall that
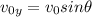`v_(0y)=v_0sin\theta`. Solving for t:

`0=(v_0sin\theta) t-(gt^2)/(2)\\(gt)/(2)=v_0sin\theta\\t=(2v_0sin\theta)/(g)\\t=(2(30(m)/(s))sin(60^\circ))/(9.8(m)/(s^2))\\t=5.30s`