Question

X(t)= 0.0945t^4 - 0.171^3 + 0.751^2 +4.53t -6.07
find the acceleration if t= 2.77s

Answer 1

The acceleration at t = 2.77 s is 7.36 m/s².

Answer:

Step-by-step explanation:

As we know that acceleration is determined by the rate of change in velocity and velocity is determined as the rate of change in distance. So indirectly, acceleration can also be determined as the second order differentiation of distance.

Such as
`a=(dv)/(dt)` and
`v=(dx)/(dt)`

So,
`a = (d^(2)x )/(dt^(2) )`

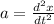
As here the x is given as function of t as
`x(t)=0.0945t^(4)-0.171t^(3)+0.751t^(2)+4.53t-6.07`

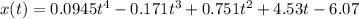
So first to determine the velocity, we have to differentiate the above function with respect to t, and the answer is

`v=0.378t^(3)- 0.513t^(2)+1.501t+4.53`

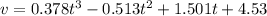
Now, to find the acceleration, we have to again differentiate this with respect to t. So the final answer is

`a=1.134t^(2)-1.026t+1.501`

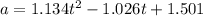
So as the time is given as t = 2.77 s, substitute this in above equation, to determine the acceleration at this time. So the answer will be

a=8.701-2.842+1.501=7.36 m/s².

Thus, the acceleration at t = 2.77 s is 7.36 m/s².