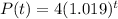Question

The world's population has grown at an average rate

of 1.9 percent per year since 1945. There were
approximately 4 billion people in the world in 1975.
Which of the following functions represents the
world's population P, in billions of people,
1 years since 1975 ? (1 billion = 1,000,000,000)
A) P(t) = 4(1.019)
B) P(t) = 4(1.9)
C) P(t) = 1.194 + 4
D) P(t) = 1.0197 +4​

Answer 1

`P(t)=4(1.019)^t`

Explanation:

we know that

The equation of a exponential growth function is equal to

`P=a(1+r)^t`

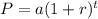
where

P ---> is the world's population

t ---> is the number of years since 1945

a ---> is the initial population in 1945

r ---> is the percent rate of growth

we have

`r=1.9\%=1.9/100=0.019`

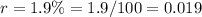
substitute

`P=a(1+0.019)^t`

`P=a(1.019)^t`

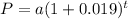
Remember that

There were approximately 4 billion people in the world in 1975

That means

Since year 1975 the initial value a=4 billion people

substitute

`P(t)=4(1.019)^t`