Answer:
 is neither significantly low nor significantly high.
is neither significantly low nor significantly high.
Explanation:
From the information given, we know that:
The mean is
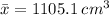 and the standard deviation is
and the standard deviation is
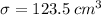 .
.
The range rule of thumb says that:
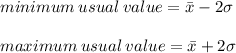
Applying these definitions, we get that
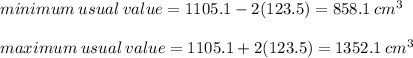
We note that
 is between
is between
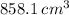 and
and
 , which indicates
, which indicates
 is neither significantly low nor significantly high.
is neither significantly low nor significantly high.