Question

For a caffeinated drink with a caffeine mass percent of 0.65% and a density of 1.00 g/mL, how many mL of the drink would be required to reach an LD50 of 180 mg/kg body mass if the person weighed 157 lb?

Answer 1

Step-by-step explanation:

First we will convert the given mass from lb to kg as follows.

157 lb =
`157 lb * (1 kg)/(2.2046 lb)`

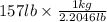
= 71.215 kg

Now, mass of caffeine required for a person of that mass at the LD50 is as follows.

`180 (mg)/(kg) * 71.215 kg`

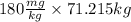
= 12818.7 mg

Convert the % of (w/w) into % (w/v) as follows.

0.65% (w/w) =
`(0.65 g)/(100 g)`

=
`(0.65 g)/(((100 g)/(1.0 g/ml)))`

=
`(0.65 g)/(100 ml)`

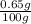
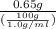
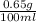
Therefore, calculate the volume which contains the amount of caffeine as follows.

12818.7 mg = 12.8187 g =
`(12.8187 g)/((0.65 g)/(100 ml))`

= 1972 ml

Thus, we can conclude that 1972 ml of the drink would be required to reach an LD50 of 180 mg/kg body mass if the person weighed 157 lb.