Question

Given two dependent random samples with the following results: Population 1 58 76 77 70 62 76 67 76 Population 2 64 69 83 60 66 84 60 81 Can it be concluded, from this data, that there is a significant difference between the two population means? Let d=(Population 1 entry)−(Population 2 entry). Use a significance level of α=0.01 for the test. Assume that both populations are normally distributed.

Answer 1

`z=(\bar d -0)/((\sigma_d)/(√(n)))=(-0.625 -0)/((6.818)/(√(8)))=-0.259`

`p_v =2*P(z<-0.259) =0.796`

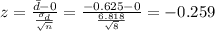
So the p value is higher than the significance level given
`\alpha=0.01`, then we can conclude that we FAIL to reject the null hypothesis that the difference mean between after and before is equal to 0. So we can conclude that we don't have significant differences between the two populations.

Explanation:

Previous concepts

Normal distribution, is a "probability distribution that is symmetric about the mean, showing that data near the mean are more frequent in occurrence than data far from the mean".

Solution to the problem

Let's put some notation :

x=values popoulation 2 , y = values population 1

x: 64 69 83 60 66 84 60 81

y: 58 76 77 70 62 76 67 76

The system of hypothesis for this case are:

Null hypothesis:
`\mu_y- \mu_x = 0`

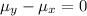
Alternative hypothesis:
`\mu_y -\mu_x \\eq 0`

The first step is calculate the difference
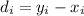`d_i=y_i-x_i` and we obtain this:

d: -6,7,-6,10,-4,-8, 7, -5

The second step is calculate the mean difference

`\bar d= (\sum_(i=1)^n d_i)/(n)= (-5)/(8)=-0.625`

The third step would be calculate the standard deviation for the differences, and we got:

`\sigma_d =(\sum_(i=1)^n (d_i -\bar d)^2)/(n) =6.818`

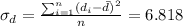
The 4 step is calculate the statistic given by :

`z=(\bar d -0)/((\sigma_d)/(√(n)))=(-0.625 -0)/((6.818)/(√(8)))=-0.259`

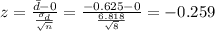
Now we can calculate the p value, since we have a two tailed test the p value is given by:

`p_v =2*P(z<-0.259) =0.796`

So the p value is higher than the significance level given
`\alpha=0.01`, then we can conclude that we FAIL to reject the null hypothesis that the difference mean between after and before is equal to 0. So we can conclude that we don't have significant differences between the two populations.