Answer:
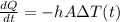
Step-by-step explanation:Newton.s law of cooling states that the rate of cooling of an object is proportional to the difference between its own temperatures and temperature of its surroundings. Mathematically,
![(dQ)/(dt) =-hA [T(t)-T(s)]\\](https://img.qammunity.org/2021/formulas/physics/high-school/n9gbp3oegde50q3ws00syxepema5q3cas0.png)
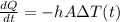
where
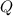 is the heat transfer
is the heat transfer
 is heat transfer coefficient
is heat transfer coefficient
 is the heat transfer surface area
is the heat transfer surface area
 is the temperature of the object's surface
is the temperature of the object's surface
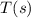 is the temperature of the surroundings
is the temperature of the surroundings