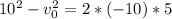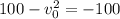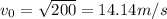Question

Nicole throws a ball straight up. Chad watches the ball from a window 5.0 m above the point where Nicole released it. The ball passes Chad on the way up, and it has a speed of 10 m/s as it passes him on the way back down. How fast did Nicole throw the ball?

Answer 1

14.14 m/s

Step-by-step explanation:

As total mechanical energy is conserved, if the potential energy is the same at Chad's position no matter if the ball is travelling up or down, then its kinetic energy, and speed, is also the same too.

Therefore, the ball would have a speed of 10m/s when it's passing Chad the 1st time. Since we know that Chad is at 5m high from the release point, we can use the following equation of motion:

`v^2 - v_0^2 = 2g\Delta s`

where v = 10 m/s is the velocity of the ball when it passes Chad, v_0 is the initial velocity of the ball when it releases, g = 10 m/s2 is the deceleration of the can, and
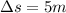`\Delta s = 5 m` is the distance traveled between Nicole and Chad. We can solve for v0:

`10^2 - v_0^2 = 2*(-10)*5`

`100 - v_0^2 = -100`

`v_0^2 = 200`

`v_0 = √(200) = 14.14 m/s`