Question

To help answer this question, Deigo wrote the division equation 3/4 divided by 2/5. Explain why this equation does not represent the situation. This is the situation After raining for 3/4 of an hour, a rain gauge is 2/5 filled. If it continues to rain at that rate at that rate for 15 more minutes, what fraction of the rain will be filled.

Answer 1

The fraction of the rain filled in the gauge will be =
`(8)/(15)`

Diego wrote the division equation incorrectly.

It must be given as
`(2)/(5)` divided by
`(3)/(4)` .

Explanation:

Given:

After
`(3)/(4)` of an hour of rain the rain gauge is
`(2)/(5)` filed.

To find the fraction of the rain that will be filled in the gauge if it continues to rain at the same rate for 15 more minutes.

Solution:

15 minutes in hours will be =
`15\ min * (1\ hour)/(60\ min)` =
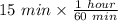`(1)/(4)` of an hour.

Given that it has rained for
`(3)/(4)` of an hour, and it continues to rain for
`(1)/(4)` of an hour more.

Thus, total time of rain will be =
`(3)/(4)+(1)/(4)=(4)/(4)=1\ hour`

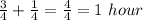
Using unitary method to find the fraction of rain filled in the gauge after 1 hour of rain.

If in
`(3)/(4)` of an hour of rain the rain gauge is
`(2)/(5)` filed.

Thus, in 1 hour of rain the gauge will be filled by =
`(2)/(5)/ (3)/(4)`

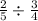
To divide fractions, we take reciprocal of the divisor and replace division by multiplication.

⇒
`(2)/(5)*(4)/(3)`

⇒
`(8)/(15)`

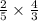
Thus, the fraction of the rain filled in the gauge will be =
`(8)/(15)`

Diego wrote the division equation incorrectly.

It must be given as
`(2)/(5)` divided by
`(3)/(4)` .