Question

Please help with question 1

Answer 1

The volume of Solid A is 120 cm³.

Explanation:

Given the area of the solids are in the ratio: 4 : 9

Let their actual areas be 4x² and 9x². We have assumed the common ratio as x² for easier computation.

We have area:
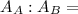`$ A_A : A_B = $` 4x² : 9x²

This implies, the length should have been of the ratio of the square root of these numbers.

Therefore, the ratios of the lengths is:
`$ √(4x^2) : √(9x^2) $`

`$ L_A : L_B = 2x : 3x $`

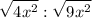
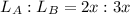
Now, volume is cube of lengths. Therefore, the ratio of volumes would be:

`$ V_A : V_B = 8x^3 : 27x^3 $`

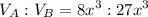
Given
`$ V_B = 405 cm^3 $`

`$ \implies 27x^3 = 405 \hspace{1mm} cm^3 $`

`$ \implies x^3 = (405)/(27) = 15 $`

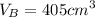
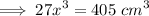
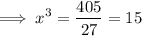
Hence, the volume of solid A,
`$ V_A = 8x^3 = 8 * 15 = \textbf{120} \hspace{1mm} cm^3 $`.

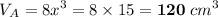Hence, the answer.