Question

One year ago Lerner and Luckmann Co. issued 15-year, noncallable, 7.5% annual coupon bonds at their par value of $1,000. Today, the market interest rate on these bonds is 5.5%. What is the current price of the bonds, given that they now have 14 years to maturity?

Answer 1

current price = $1191.79

Step-by-step explanation:

given data

time t = 15 year

annual coupon bonds rate = = 7.5 %

par value = $1000

interest rate = 5.5%

maturity time = 14 year

to find out

current price of the bonds

solution

we get here first annual coupon rate = 7.5% of 1000

annual coupon rate C = $75

so now we get current price of bond

current price of the bonds =
`(C)/((1+r)) +(C)/((1+r)^2) +(C)/((1+r)^3) +(C)/((1+r)^4) ..........(C)/((1+r)^(13)) + (C+par\ value)/((1+r)^(14))` .................1

put here value

current price =
`(75)/((1+r)) +(75)/((1+r)^2) +(75)/((1+r)^3) +(75)/((1+r)^4) ..........(75)/((1+r)^(13)) + (75+1000)/((1+r)^(14))`

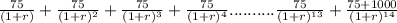
current price =
`(75)/((1+r)) (1-((1)/(1+r))^(14) )/(r) (1+r) + (1000)/((1+r)^(14))`

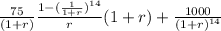
solve it we get

current price = $1191.79