Question

Cylinders A and B are similar solids. The base of cylinder A has a circumference of 4r units. The base of cylinder B has an

area of 9. units
The dimensions of cylinder A are multiplied by what factor to produce the corresponding dimensions of cylinder B?

Answer 1

C 3/2

Explanation:

Answer 2

Question:

Cylinders A and B are similar solids. The base of cylinder A has a circumference of 4π units. The base of cylinder B has an area of 9π units.The dimensions of cylinder A are multiplied by what factor to produce the corresponding dimensions of cylinder B?

Answer:

Dimensions of cylinder A are multiplied by
`(3)/(2)` to produce the corresponding dimensions of cylinder B

Solution:

Given that, Cylinders A and B are similar solids

The base of cylinder A has a circumference of
`4 \pi` units

The formula for the circumference of a circle is:

`C = 2 \pi r`

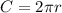
where, "r" is the radius of circle

`4 \pi = 2 \pi r`

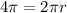
r = 2

Thus, radius of cylinder A = 2 units

The base of cylinder B has an area of
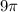`9 \pi` units

The area of circle is given by formula:

`Area = \pi r^2`

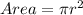
where, "r" is the radius of circle

`9 \pi = \pi r^2\\\\r^2 = 9\\\\r = 3`

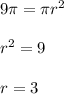
Thus radius of cylinder B is 3 units

Let the multiplication factor be "x"

From given,

The dimensions of cylinder A are multiplied by what factor to produce the corresponding dimensions of cylinder B

Therefore,

`Radius\ of\ Cylinder\ A = x * Radius\ of\ Cylinder\ B\\\\2 * x = 3\\\\x = (3)/(2)`

Thus dimensions of cylinder A are multiplied by
`(3)/(2)` to produce the corresponding dimensions of cylinder B