Answer:
140 ounces of water and 105 ounces of 35% salt solution.
Step-by-step explanation:
Let
 be the ounces of water that need to be added and
be the ounces of water that need to be added and
 be the ounces of 35% solution that need to to be added.
be the ounces of 35% solution that need to to be added.
We know that the total of ounces of water and of 35% solution must be 245 ounces:

35% of
 is the salt, and since no salt is added with addition of
is the salt, and since no salt is added with addition of
 liters of water, this must be equal to the amount of salt in the final 15% solution:
liters of water, this must be equal to the amount of salt in the final 15% solution:
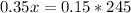
we solve for
 and get:
and get:
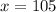
We put this value into
 and solve for
and solve for
 to get:
to get:
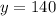
Thus we have 140 ounces of water and 105 ounces of 35% solution.