Question

a. A beam of light is incident from air on the surface of a liquid. If the angle of incidence is 26.7° and the angle of refraction is 18.3°, Find the critical angle for the liquid when surrounded by air?b. A light ray, traveling in air, strikes the surface of abeaker of mineral oil at an angle of 37.5° with thenormal to the surface. The speed of light in mineral oil is 2.17 x10^8 m/s.. Calculate the angle of refraction.

Answer 1

a) Angle of incidence i = 26.7°

Angle of refraction r = 18.3°

From Snell’s law index of refraction of the liquid

`n = (sin\ i)/(sin\ r)`

`n = (sin\ 26.7^0)/(sin\ 18.3^0)`

n = 1.43

So, critical angle

`C= sin^(-1)((1)/(n))`

`C= sin^(-1)((1)/(1.43))`

C = 44.33°

b) Angle of incidence, i = 37.5°

speed of light in mineral oil , v = 2.17 x 10⁸ m/s

speed of light in air, c = 3 x 10⁸ m/s

refractive index of the oil

`n = (c)/(v)`

`n = (3* 10^8)/(2.17* 10^8)`

n = 1.38

again using Snell's law

`n = (sin\ i)/(sin\ r)`

`sin\ r = (sin\ i)/(n)`

`sin\ r = (sin\ 37.5^0)/(1.38)`

`r = sin^(-1)(0.441)`

r = 26.18°

hence, the angle of refraction is equal to r = 26.18°

Answer 2

(a). The critical angle for the liquid when surrounded by air is 44.37°

(b). The angle of refraction is 26.17°.

Step-by-step explanation:

Given that,

Incidence angle = 26.7°

Refraction angle = 18.3°

(a). We need to calculate the refraction of liquid

Using Snell's law

`n=(\sin i)/(\sin r)`

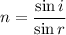
Put the value into the formula

`n=(\sin 26.7)/(\sin 18.3)`

`n=1.43`

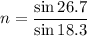
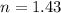
We need to critical angle for the liquid when surrounded by air

Using formula of critical angle

`C=\sin^(-1)((1)/(n))`

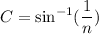
Put the value into the formula

`C=\sin^(-1)((1)/(1.43))`

`C=44.37^(\circ)`

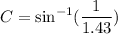
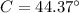
(b). Given that,

Incidence angle = 37.5°

Speed of light in mineral
`v=2.17*10^(8)\ m/s`

We need to calculate the index of refraction

Using formula of index of refraction

`n=(c)/(v)`

Put the value into the formula

`n=(3*10^(8))/(2.17*10^(8))`

`n=1.38`

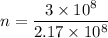
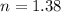
We need to calculate the angle of refraction

Using Snell's law

`n=(\sin i)/(\sin r)`

`\sin r=(\sin i)/(n)`

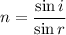
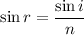
Put the value into the formula

`\sin r=(\sin 37.5)/(1.38)`

`r=\sin^(-1)((\sin 37.5)/(1.38))`

`r=26.17^(\circ)`

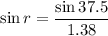
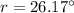
Hence, (a). The critical angle for the liquid when surrounded by air is 44.37°

(b). The angle of refraction is 26.17°.