Answer:
311.25k
Step-by-step explanation:
The question assumes heat is not lost to the surroundings, therefore
heat emitted from hotter sample (
 )= heat absorbed by the less hotter sample(
)= heat absorbed by the less hotter sample(
 )
)
The relationship between heat (q), mass (m) and temperature (t) is
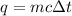
where c is specific heat capacity,
 temperature change.
temperature change.
 =
=
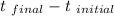
equating both heat emitted and absorb
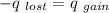
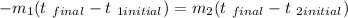
where the values with subset 1 are the values of the hotter sample of water and the values with subset 2 are the values of the less hot sample of water.
C will cancel out since both are water and they have the same specific heat capacity.
so we have
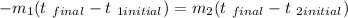
where m1 = 50g, t 1initial = 330, m2 = 30g, t2 initial = 280,t final (final temperature of the mixture) = ?
-50 * (
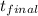 - 330) = 30 * (
- 330) = 30 * (
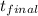 - 280)
- 280)
-50
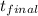 + 16500 = 30
+ 16500 = 30
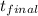 - 8400
- 8400
80
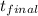 = 16500+8400
= 16500+8400
80
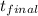 = 24900
= 24900
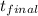 = 24900/80 = 311.25k
= 24900/80 = 311.25k