Question

Refer to the accompanying​ TI-83/84 Plus calculator display of a​ 95% confidence interval. The sample display results from using a simple random sample of the amounts of tar​ (in milligrams) in cigarettes that are all king​ size, nonfiltered,​ nonmenthol, and​ non-light. Express the confidence interval in the format of x overbarplus or minusE. ZInterval ​(21.182​,23.958​) x overbarequals22.57 nequals25 The confidence interval is nothingplus or minus nothing.

Answer 1

[22.57 ± 2.776]

Explanation:

Hello!

You have the 95% Confidence Z-interval (21.182;23.958), the mean X[bar]= 22.57 and the sample size n=25.

The formula for the Z interval is

[X[bar] ±
`Z_(1-\alpha /2) *( (Sigma)/(√(n) ) )`]

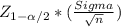The value of Z comes from tha standard normal table:

`Z_(1-\alpha /2) = Z_(0.975)= 1.96`

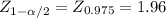
The semiamplitude (d) or margin of error (E) of the interval is:

E or d= (Upperbond- Lowerbond)/2 = (23.958-21.182)/2 = 2.776

[X[bar] ± E]

[22.57 ± 2.776]

I hope it helps!