Answer:
95.85
Explanation:
The area A of a circle as a function of its radius r is given by πr².
So, A(r) = πr²
Now, if there is a function f(x) and we have to calculate the average rate of change between the interval x = a and x = b is given by
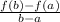
Now, A(5.5) = π(5.5)² = 95.07 and A(25) = π(25)² = 1964.28
So, the average rate of change in area between the intervals r = 5.5 to r = 25 will be given by
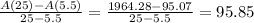 (Answer)
(Answer)